For the Math: Fortune's Favor
- PDH Home Base

- May 2, 2018
- 15 min read

Today is special for me. Today, is a day where I do something new. For any fellow content creators out there, you all can sympathize with what is known as "writer's block." For some time now, I've been in a bit of a creativity rut. Drying up on ideas, seeing fewer angles, and not putting out my best stuff. For those of you who have been a part of our growing community, I wanted to do better. I wanted to try something new, something different. For the first time, I'm going to take a step outside of PDH.
Before I jump into, I have to tell you about myself, and my background. First of all, although PDH is my passion, it isn't the only format that I play. I feel quite comfortable assuming that I am not the only person in our community that enjoys several formats, but I don't want to get too far from our goal here. I'm staying within the world of casual / budget play on this piece.
The other thing about, is that I have a relatively strong background in Mathematics. Nowadays, it is rare that I have a chance to explore this other field of study, let alone find a way to bring math into my favorite game. it's with this point, that I discovered my new angle. Among my casual browsing of cards, I started coming across cards that create these single-round "sub-games" within the game itself. These are games that have a very specific way to be played, and math can explain exactly how to approach these fun cards.

Let's introduce the card of the hour. Fortune's Favor acts very similar to Fact or Fiction, but adds one very special element to this situation. Choice A) A value you can see. Choice B) A mystery. Which pile do you pick?
In this article, with support of Mathematics and Economic Game Theory, I will propose my theory to you as to how this card should be played. I will show how the caster of this spell should pick the pile, and how the sorter of the two piles should create these two piles.
The first thing that I need to do before making any sort of proof, is to establish a set of assumptions that I will use to support my claim, as well as a symbol legend for simplifying my writing. For those reading that are less familiar with math proofs, it is important to establish a starting point, and some structured ground to build upon. After I present my case, then I will observe the assumptions, and see whether or not any of them could be violated, and how to adjust the proposition accordingly.
Legend and Assumptions
{L = Library} {H = Hand, H is a subset of L} {P = Pile Cards = A, B, C, D | P is a subset of L}
Two Players: Caster, {U(+) = Utility received from +} {FU = Face Up}
Sorter {FD = Face Down}
1) "Utility that the Caster receives from each of the 4 cards are weakly dominant of one another." You get 4 cards. We are assuming that all of these cards will give the caster value. Furthermore, we also assume that we can put all 4 of these cards in order, from least to most valuable.
=> U(A) < U(B) < U(C) < U(D)
2) "All players are rational." We are going to assume that all decisions people make are strictly strategy based, and there will be no emotional intervention.
3) "All four cards are independent of one another." We are stating that among the 4 cards, none of them have any synergy among themselves. Furthermore, there are no combo pieces in the pile.
4) "All players are knowledgeable of the game, and the cards being used."
5) "The Caster and the Sorter are the only two relevant players in this game." In other words, nobody else receives any information from observing this game, nor will other players influence the choices made.
Establishing the Goals and Payoffs
In this game, it is absolutely essential to assess the goals of both the caster, and the sorter. I'm going to do my best to use as little mathematical jargon as possible to lay this out.
The Caster, no matter what, will receive two cards from this arrangement. The goal of the caster is to receive the most utility possible, given the two piles provided. The sorter, on the other hand, needs to create two piles, in hopes of minimizing the utility the caster receives. The Sorter, however, has something to keep in mind. They must make a decision to minimize the caster's utility, without knowing which pile will be picked.
One of the most important claims that I will make in this entire article is simple. The Sorter "cannot" accurately guess which pile will be picked. In fact, to make such an assumption risks giving the caster the highest utility possible, hence the sorter failing. How can this be?
Recall our second assumption. This will be the foundation of this idea. The decision made will not be based off emotion. That means that whatever emotional trigger the Face Up pile creates, will not have any effect on the decision. So, what does control which pile the caster picks?
Recalling assumptions 1 and 4, we can place the 4 cards in order from least to most valuable. Is there potential that two of the cards are of identical value? Absolutely. Is it possible that you could make two piles of equal value? The answer to this question is also "yes." However, despite the possibility, we will observe later on that such identical piles are unlikely. So, no matter how small the difference, we can fairly assume one pile has more worth than the other. (For what I will present below, you see that even if the piles are identical, it does not change the below case).
Suppose the sorter has made pile 1, and pile 2, where one is weakly dominant of the other. The sorter must decide which will be [FU] and [FD]. The caster can see [FU] but [FD] still completely unknown. The question, which pile is the larger of the two? Let's observe two cases, and notice how the final result is the same.
Case 1:
i) The Sorter chooses for [FU] to be the larger pile.
ii) The Caster assumes that [FU] is larger, due to what can be observed.
iii) Sorter observes ii, so chooses to make [FD] to be larger.
iv) Caster observes ii and iii, so decides on choosing [FD].
v) Sorter observes iv, and switches back to [FU].
vi) Caster observes v, and decides to choose [FU].
This exchange of movements will continue indefinitely. We are safe to assume this, due to assumption 2. By assuming rationality, we can also the assume that both the caster and the sorter each assume the other to be rational. Without any emotional intervention, there is no way for this indecisive cycle to end. With that being said, suppose we graphed this movement in decision making.
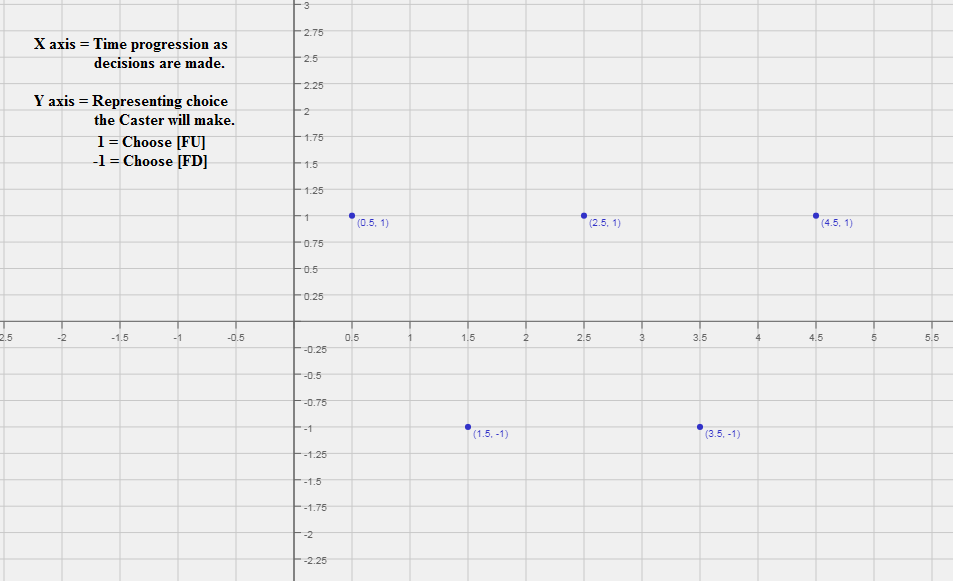
Graphing the first few movements of decisions for the Caster seems to appear rather bland, but actually gives us a great deal of information. Suppose we attempted to fit a function over these point, to better understand the movement of decision.
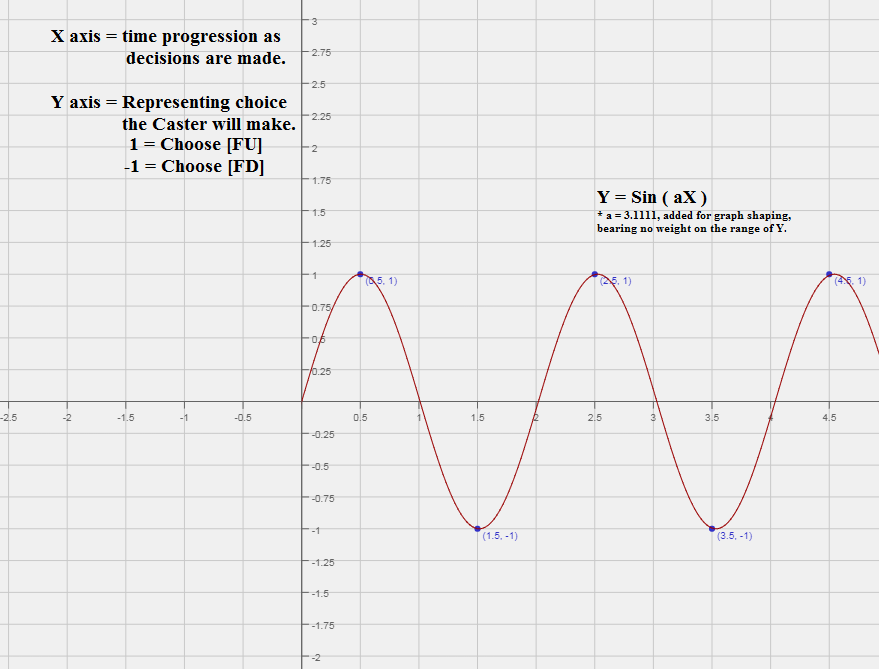
We can see that we very much can fit a function over these points. Furthermore, we can now observe what decision the caster will make as time goes on indefinitely. Or can we?

With the help of Wolfram Alpha, we can see that as time goes on indefinitely, there is no specific number that it approaches. There is no decisive answer as to what decision the caster will make as time progresses indefinitely. For all we can tell, one decision is as equally likely as the other. This is case 1, but what about case 2?
Case 2:
i) The Sorter chooses for [FD] to be the larger pile.
ii) The Caster assumes that [FD] is larger, due to what can be observed.
iii) Sorter observes ii, so chooses to make [FU] to be larger.
iv) Caster observes ii and iii, so decides on choosing [FU].
v) Sorter observes iv, and switches back to [FD].
vi) Caster observes v, and decides to choose [FD].
As you can see, the progression of movements begins slightly different, but as time goes on, the transition of decisions becomes identical. Suppose we decided to graph this progression of movements. What do you suppose we will see?
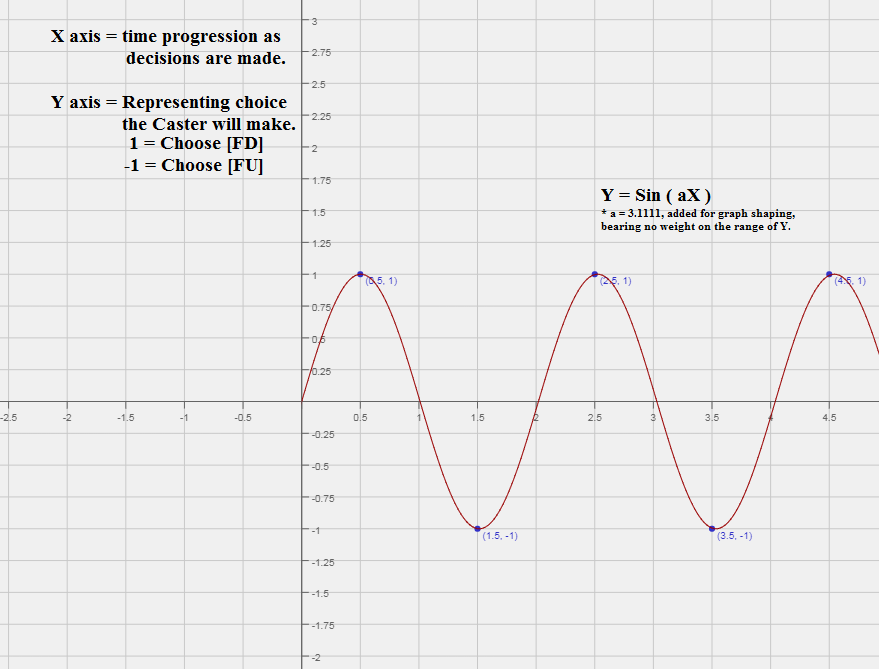
Not to our surprise, we get the exact same plotting of points, with the exact same function, with the same results as time goes on indefinitely. In both cases, the Caster never decides on a decision, if rational thought alone are considered. If a choice is made, they will make this choice at random.
The Sorter's Methods
With an understanding of the payoffs for both parties, and understanding how the Caster is obligated to proceed, that leaves a very interesting question in regards to the Sorter. If the caster makes their decision entirely at random, how does the sorter design the two piles? How much value is placed into each pile, and which of the two does the sorter choose to expose?
As I had earlier stated above, the sorter is attempting limit the amount of utility the caster receives, regardless of the choice the caster makes. There is no way to predict which choice is made, and where each choice is equally likely, the caster choosing the most valuable pile is equally as probable as picking the lesser valued pile. The sorter must minimize their risk, and therefore, must hedge.
U(A) = X , X = V(X) + K(X) , V: Utility that we can observe.
U(B) = X + Y , Y = V(Y) + K(Y)
U(C) = X + Y + Z , Z = V(Z) + K(Z) , K: Utility that cannot be observed.
U(D) = X + Y + Z + W , W = V(W) + K(W)
Every card has a value that can be very closely estimated, an assumption safe to make by recalling Assumption 4. The utility of each card is weakly dominant or inferior to another, hence allowing us to sort them in order. This is how we were able to break down how to value each card, as seen above. I said that we can estimate very close to the exact value, but we cannot be perfectly accurate. The reason for this, is that we cannot be sure of interaction with either the Caster's hand, or the remaining cards in the library. Despite this, we can get close, very close.
How must the sorter make the piles? The goal is to make the piles as close to the same value as possible. We cannot control which pile is picked, and if the largest pile is picked, the sorter fails. The piles need to be close in value.
A & B = 2X + Y A & C = 2X + Y + Z A & D = 2X + Y + Z + W
C & D = 2X + 2Y 2Z + W B & D = 2X + 2Y + Z + W B & C = 2X + 2Y + Z
Diff 1 = | Y + 2Z + W | Diff 2 = | Y + Z | Diff 3 = | Y - Z |
Observing Utility differences between piles, it is apparent that Diff 3 is most likely to be the smallest value. The sorter will be required to pair card A with D, and card B and C. We know how to make the piles, but which is which? Which pile is face up, and which is face down? Recalling Assumption 5, if the Caster and the Sorter are the only two relevant players to this game, then it actually doesn't matter. The decision must be made at random.
The Simple Play
Given that the 5 assumptions hold, and I have established reasoning for all decisions, it is now necessary to lay out the basic layout of how the Caster and the Sorter will play this game. I'm going to use an example to lay out the play. Suppose the Sorter is given the 4 cards below:

An adequate display of some of my favorite blue cards in EDH, let's suppose these are the 4 cards that the sorter is given. The Sorter understands that they cannot predict the Caster's decision, so they must design the piles in accordance to the uncertainty. The sorter must create Pile 1 with cards A and D, and create Pile 2 with cards B and C.

In this case, these are the two piles that must be made. The following decision the sorter must make will be in regards to which pile is which. Such a decision will be made at random. Due to the inability to predict the decision of the caster, the Sorter will choose the Face Down pile at random.
The Caster, knowing exactly how the Sorter has played this game, also knows that there is no certainty as to which pile is of greater value. The Caster will also pick either Face Up, or Face Down, at random.
Violating the Assumptions
For you seasoned players who have been following this so far, I can imagine that there must be areas in which you disagree with my proposition. I am not just comfortable, but certain of the proposition above, given the 5 assumptions hold. However, that's the issue here with all of this. Assumptions are a very dangerous thing to make. I used assumptions to build this proposition, so let us examine how it holds up, once the assumptions are violated.
The 1st Assumption: Utility that the Caster (K) receives from each of the 4 cards are weakly
dominant of one another.
=> U(A) < U(B) < U(C) < U(D)
Although the 4th assumption is required in order to assume the sorter can recognize the value of the cards, the statement itself is independent. Suppose the above wasn't true, and that none of the cards were at the least weakly dominant of one another. That leave only two other cases: Strictly dominant, or equal. If all 4 cards are strictly dominant of one another, they can still be ordered, as seen above. If all of the cards are equal, then this whole scenario no longer matter, because which combinations the sorter creates, they will be the exact same. This assumption is safe to use.
The 2nd Assumption: All players are rational.
Suppose the Sorter is irrational, and allows a sense of taking a risk to decide how to make the piles. The caster is unaware of this risk being taken, and will proceed as normal. That now places the sorter in a 50% risk of handing the highest value to the opponent.
Suppose the Caster is irrational, and allows the allure of the shown pile to influence their decision to either accept, or risk the hidden pile. The Sorter has approached these two piles as normal, so the total value given to the caster is hedged, regardless. We can allow this assumption to hold.
The 3rd Assumption: "All four cards are independent of one another."
Understanding how many decks are played, the possibility of this is not out of the question. In the event that two cards in a pile that do have synergy with each other, it is less than great for the Caster, but fantastic for the Sorter.
Let me explain. Recalling above, I broke down the utility of each card into two parts. There is the value that can be observed, and the value that cannot be observed. What specifically is it that we cannot observe? The cards in the Caster's hand, and the cards in their library. This lack of knowledge creates an uncertainty as to which pile is truly larger. However, in the event that two cards in the pile create synergy, this actually removes that uncertainty, and tells you the exact value (or at least a fair amount of the unobserved value K) of the cards.

For this example, I'll use some of my favorite black EDH cards. If synergy is not taken into account, this is how the cards would be ordered. However, for any seasoned EDH player, they do not need to read the cards to recognize the lethal combo above. Using our above method would be incorrect, but by observing the true value of the cards, they are now both worth more than Sorin Markov, and need to be separated, in order to hedge.
Regardless of this potential scenario, the caster has no information, indicating that this scenario has indeed occurred, so they have no information, nor incentive, to behave differently. Despite the violation in the assumption, the outcome remains the same, so this assumption is still safe to make.
The 4th Assumption: All players are knowledgeable of the game, and the cards being used.
Suppose one or both players are indeed lacking knowledge of the cards, as well as the game. Despite a player's inexperience, there is no incentive for either player to assume their opponent is also inexperienced. There is no way to make a dominant play if a player doesn't have the knowledge of the game to make the best move. Rationality is irrelevant to this matter.
This assumption is safe to make, because in most cases, it is dangerous to assume the opposite. Going into a game, If you assume your opponent to be knowledgeable, and this proves to be incorrect, this is an advantage. There is no reason to change the method of play, so this assumption may hold.
The Multiplayer Problem
After observation of the first 4 assumptions, it is clear to see that a violation of any one of them will not cause an issue with the proposed method above. However, the 5th assumption is much more complicated, and will make an impact on the decisions of both the Caster and the Sorter.
The 5th Assumption: The Caster and the Sorter are the only two relevant players in this game.
Suppose this exchange between the Caster and Sorter is taking place during a 4-player game. For convenience, let's refer to these two players as the Observers (Observers 1 and 2). The 5th assumption states that no individuals watching the Sorter or Caster will have any impact on the game. However, it is unfortunately not so simple. The Observers can see the cards in the Face Up pile, and will see what cards are discarded. If the caster chooses the Face Up pile, the observers are now aware of what is in their hand. In the event the Face Down pile is chosen, the observers are now missing information. It is for this reason, that decisions are no longer made at random, thus altering the above proposed solution.
Very simply put, our proposition no longer applies. However, that does not mean we must dispose of it entirely. In fact, the majority of this can remain. The important part for us is to understand what changes, and why.

Here is a replication of our first example above. The nature of how the piles have been designed hasn't changed, but how the sorter picks which pile is which is no longer at random. The question is, why?
Recall the primary goal of the sorter. The goal is to limit the amount of utility given to the caster, regardless of the decision the caster makes. Despite the change in circumstances, there still is a degree of uncertainty as to which pile the caster will pick. There is still an amount of unknown knowledge for each card, so the sorter cannot know the true value of each pile. There can only be a close estimation as to which is larger.
Suppose, in this circumstance, the sorter has concluded the pile consisting of Cyclonic Rift to be the larger valued of the two, and has chosen to set is as Face Up. This decision, unlike the two player case, was not random. The caster may be able to observe the largest valued pile (and is aware of this), but so can the observers. This means that if the caster chooses the Face up pile, three opponents are aware of what is in their hand. This reduces the value of this pile, because now as opposed to one, there are three opponents that can respond to this premeditated threat.
Here is where the appeal of the face down pile comes into play. If the caster chooses the face down pile, the two observers are missing information. Due to elements of uncertainty throughout this solution, the caster can indeed count on the two piles being created with a similar value, with the larger of the two face up.
Which pile the Caster chooses to pick, comes down to what cards remain in their hand, and to what degree their opponents can respond to the threat of the face up pile. This is all information that the sorter cannot observe, so this is the most economic play, given the circumstance.
Who is who?
This shows how the game is played, once roles are assumed, but there is one more element of this game that must be observed. The caster is responsible for choosing who of their three opponents will be the sorter. Although not seeming to be such a critical decision, there is an impact to the balance of this game.
Recalling the 4th assumption, the caster is not only knowledgeable of their own cards, but also the general strengths and weaknesses of their opponents' decks. If the caster wishes to have a higher probability of certainty, the opponent seen as the weakest threat must be chosen.
This is the correct choice for several reasons. Recall one of the positives for the sorter to show the larger of the two piles. There is a chance that the sorter cannot deal with the more dangerous of the threats, and will rely on an observer to respond. Furthermore, if the caster chooses to take the face down pile, the player least likely to remove such threats will be the only one with knowledge of said threats. The caster must pick their weakest ally.
The 6th Assumption
With all cases observed, there is but one final assumption to be discussed. It may seem as if such a statement is unnecessary, but for those that know the politics of the game, it is indeed quite necessary.
The 6th Assumption: All relevant players in the game are strictly opponents.
Such a statement sounds unusual, but suppose that this statement is violated. Suppose this "game" is now impacted by "alliances" or "fixed arrangements." In this case, this game is no longer a game, but simply an interaction. This entire proposition is surrounded around how to play this game. If the 6th assumption is violated, then everything above is no longer relevant.
In conclusion, without repetition, let the above show my proposition for the ideal play of the ever so interesting "Fortune's Favor." If you are mathematically inclined or have interest in such games, and wish to either to discuss, or challenge and disprove my proposition, please feel free to contact me at pdhhomebase@gmail.com. As I discover more cards of such mathematical interest over time, I intend to explore the solutions to such games.
I do not intend to make a habit of moving beyond PDH, as our passions live with our format. With that being said, there is a lot to be said about a game that can be played in so many different ways, and present unique, and quality experiences. When such special circumstances arise that can be discussed, I don't wish to stay confined, as this piece has helped me break my writers block. Let us all never lose our sense of enjoyment and curiosity, and never lose our sense of exploration.
-Kyle
@PDH_Homebase

header.all-comments